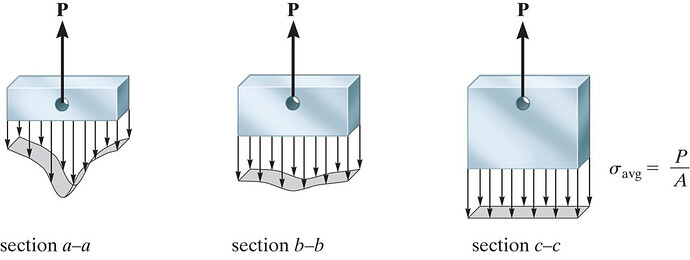
According to Saint-Vernant’s principle, stress concentrations even out when one moves away from where the force or boundary condition is applied. A good estimation to assume the “flattening” of stress peaks is one critical dimension, which in case of a rod or pipe is the diameter of the rod. This is shown in the figure below, where the critical dimension of a rectangular rod is the diagonal of the cross-section:
This means that for model that are symmetrical except for some small details, we can take advantage of symmetry by only modelling half of the model. Bear in mind that in a case like this, you will not be able to capture local influences due to the asymmetry. If however the area of interest is at least one critical dimension away from the asymmetry, then we can split the model.